Partial derivatives of 3 variables. First order partial derivatives. Full differential. Similarly, we obtain the partial increment of z over y

Functions of two variables, partial derivatives, differentials and gradient

Topic 5.Functions of two variables.

partial derivatives

Definition of a function of two variables, methods of setting.

Partial derivatives.

Gradient of a function of one variable

Finding the largest and smallest values ​​of a function of two variables in a closed bounded domain

1. Definition of a function of several variables, methods of setting

For functions of two variables
domain of definition is some set of points on a plane
, and the range of values ​​is the interval on the axis
.

For visual representation functions of two changes nyh are applied level lines.

Example . For function
build a graph and level lines. Write down the equation of the level line passing through the point
.

Graph of a linear function is plane in space.

For a function, the graph is a plane passing through the points
,
,
.

Function level lines are parallel lines whose equation is
.

For linear function of two variables
level lines are given by the equation
and represent a family of parallel lines on a plane.

4

Graph of a function 0 1 2 X

Function level lines

Private projectsderived functions of two variables

Consider the function
. Let's give the variable at the point
arbitrary increment
, leaving variable value unchanged. Corresponding Function Increment

called private increment of a function by variable at the point
.

Defined similarly partial function incrementby variable: .

Designationpartial derivative with respect to: , ,
,
.

Partial derivative of a function with respect to a variable called the final limit :

Designations: , ,
,
.

To find the partial derivative
by variable, the rules for differentiating a function of one variable are used, assuming the variable is constant..

Similarly, to find the partial derivative with respect to a variable a variable is considered constant .

Example . For function
find partial derivatives
,
and calculate their values ​​at the point
.

Partial derivative of a function
by variable is under the assumption that it is constant:

Let us find the partial derivative of the function with respect to , assuming constant:

Let us calculate the values ​​of partial derivatives at
,
:

;
.

Second order partial derivatives functions of several variables are called partial derivatives of first order partial derivatives.

Let us write down the 2nd order partial derivatives for the function:

;
;

;
.

;
etc.

If mixed partial derivatives of functions of several variables are continuous at some point
, then they equal to each other at this point. This means that for a function of two variables, the values ​​of mixed partial derivatives do not depend on the order of differentiation:

.

Example. For the function, find the second order partial derivatives
And
.

Solution

The mixed partial derivative is found by successively differentiating first the function by (assuming constant), then differentiating the derivative
by (considering constant).

The derivative is found by first differentiating the function with respect to , then the derivative with respect to .

Mixed partial derivatives are equal to each other:
.

3. Gradient of a function of two variables

Gradient Properties

Example . Given a function
. Find the gradient
at the point
and build it.

Solution

Let's find the coordinates of the gradient - partial derivatives.

At the point
gradient equal to . Beginning of the vector
at point , and the end at point .

5

4. Finding the largest and smallest values ​​of a function of two variables in a closed limited area

Formulation of the problem. Let there be a closed bounded region on the plane
is given by a system of inequalities of the form
. It is required to find points in the region at which the function takes the largest and smallest values.

Important is problem of finding an extremum, the mathematical model of which contains linear restrictions (equations, inequalities) and linear function
.

Formulation of the problem. Find the largest and smallest values ​​of a function
(2.1)

under restrictions

(2.2)

. (2.3)

Since there are no critical points for a linear function of many variables inside region
, then the optimal solution, which delivers an extremum to the objective function, is achieved only on the border of the region. For a region defined by linear constraints, the points of possible extremum are corner points. This allows us to consider the solution to the problem graphical method.

Graphical solution of a system of linear inequalities

To solve this problem graphically, you must be able to solve graphically systems of linear inequalities with two variables.

Procedure:

Note that the inequality
defines right coordinate half-plane(from axis
), and the inequality
- upper coordinate half-plane(from axis
).

Example. Solve graphically the inequality
.

Let us write down the equation of the boundary line
and build it based on two points, for example,
And
. A straight line divides a plane into two half-planes.

Point coordinates
satisfy the inequality (
– true), which means that the coordinates of all points of the half-plane containing the point satisfy the inequality. The solution to the inequality will be the coordinates of the points of the half-plane located to the right of the boundary line, including points on the boundary. The desired half-plane is highlighted in the figure.

Solution
system of inequalities is called acceptable, if its coordinates are non-negative, . The set of feasible solutions to the system of inequalities forms a region that is located in the first quarter of the coordinate plane.

Example. Construct the solution domain of the system of inequalities

The solutions to the inequalities are:

1)
- half-plane located to the left and below relative to the straight line ( )
;

2)
– half-plane located in the lower-right half-plane relative to the straight line ( )
;

3)
- half-plane located to the right of the straight line ( )
;

4) - half-plane above the x-axis, that is, straight line ( )
.

0

Range of feasible solutions of a given system of linear inequalities is a set of points located inside and on the boundary of the quadrilateral
, which is intersection four half-planes.

Geometric representation of a linear function

(level lines and gradient)

Let's fix the value
, we get the equation
, which geometrically defines a straight line. At each point on the line the function takes the value and is level line. Giving different meanings, For example,

, ... , we get a lot of level lines - set of parallel direct.

Let's build gradient- vector
, whose coordinates are equal to the values ​​of the coefficients of the variables in the function
. This vector: 1) perpendicular to each straight line (level line)
; 2) shows the direction of increase of the objective function.

Example . Plot level lines and gradient functions
.

Level lines at , , are straight

,
,

, parallel to each other. The gradient is a vector perpendicular to each level line.

Graphically finding the largest and smallest values ​​of a linear function in an area

Geometric formulation of the problem. Find in the solution domain of the system of linear inequalities the point through which the level line passes, corresponding to the largest (smallest) value of a linear function with two variables.

Sequencing:

4. Find the coordinates of point A by solving the system of equations of lines intersecting at point A, and calculate the smallest value of the function
. Likewise for point B and highest value functions
. built on points.variables Privatederivativesfunctions several variables and differentiation technique. Extremum functionstwovariables and its necessary...

Each partial derivative (by x and by y) of a function of two variables is the ordinary derivative of a function of one variable for a fixed value of the other variable:

(Where y= const),

(Where x= const).

Therefore, partial derivatives are calculated using formulas and rules for calculating derivatives of functions of one variable, while considering the other variable constant.

If you do not need an analysis of examples and the minimum theory required for this, but only need a solution to your problem, then go to online partial derivative calculator .

If it’s hard to concentrate to keep track of where the constant is in the function, then in the draft solution of the example, instead of a variable with a fixed value, you can substitute any number - then you can quickly calculate the partial derivative as the ordinary derivative of a function of one variable. You just need to remember to return the constant (a variable with a fixed value) to its place when finishing the final design.

The property of partial derivatives described above follows from the definition of a partial derivative, which can be found in exam questions. Therefore, to familiarize yourself with the definition below, you can open the theoretical reference.

Concept of continuity of function z= f(x, y) at a point is defined similarly to this concept for a function of one variable.

Function z = f(x, y) is called continuous at a point if

Difference (2) is called the total increment of the function z(it is obtained as a result of increments of both arguments).

Let the function be given z= f(x, y) and period

If the function change z occurs when only one of the arguments changes, for example, x, with a fixed value of another argument y, then the function will receive an increment

called partial increment of function f(x, y) By x.

Considering a function change z depending on changing only one of the arguments, we effectively change to a function of one variable.

If there is a finite limit

then it is called the partial derivative of the function f(x, y) by argument x and is indicated by one of the symbols

(4)

The partial increment is determined similarly z By y:

and partial derivative f(x, y) By y:

(6)

Example 1.

Solution. We find the partial derivative with respect to the variable "x":

(y fixed);

We find the partial derivative with respect to the variable "y":

(x fixed).

As you can see, it does not matter to what extent the variable is fixed: in this case it is simply a certain number that is a factor (as in the case of the ordinary derivative) of the variable with which we find the partial derivative. If the fixed variable is not multiplied by the variable with which we find the partial derivative, then this lonely constant, no matter to what extent, as in the case of the ordinary derivative, vanishes.

Example 2. Given a function

Find partial derivatives

(by X) and (by Y) and calculate their values ​​at the point A (1; 2).

Solution. At fixed y the derivative of the first term is found as the derivative of the power function ( table of derivative functions of one variable):

.

At fixed x the derivative of the first term is found as the derivative of the exponential function, and the second - as the derivative of a constant:

Now let's calculate the values ​​of these partial derivatives at the point A (1; 2):

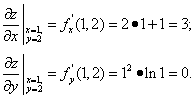
You can check the solution to partial derivative problems at online partial derivative calculator .

Example 3. Find partial derivatives of a function

Solution. In one step we find

(y x, as if the argument of sine were 5 x: in the same way, 5 appears before the function sign);

(x is fixed and is in this case a multiplier at y).

You can check the solution to partial derivative problems at online partial derivative calculator .

The partial derivatives of a function of three or more variables are defined similarly.

If each set of values ​​( x; y; ...; t) independent variables from the set D corresponds to one specific value u from many E, That u called a function of variables x, y, ..., t and denote u= f(x, y, ..., t).

For functions of three or more variables, there is no geometric interpretation.

Partial derivatives of a function of several variables are also determined and calculated under the assumption that only one of the independent variables changes, while the others are fixed.

Example 4. Find partial derivatives of a function

.

Solution. y And z fixed:

x And z fixed:

x And y fixed:

Find partial derivatives yourself and then look at the solutions

Example 5.

Example 6. Find partial derivatives of a function.

The partial derivative of a function of several variables has the same mechanical meaning is the same as the derivative of a function of one variable, is the rate of change of the function relative to a change in one of the arguments.

Example 8. Quantitative value of flow P railway passengers can be expressed by the function

Where P– number of passengers, N– number of residents of correspondent points, R– distance between points.

Partial derivative of a function P By R, equal

shows that the decrease in passenger flow is inversely proportional to the square of the distance between corresponding points with the same number of residents in points.

Partial derivative P By N, equal

shows that the increase in passenger flow is proportional to twice the number of residents of settlements at the same distance between points.

You can check the solution to partial derivative problems at online partial derivative calculator .

Full differential

The product of a partial derivative and the increment of the corresponding independent variable is called a partial differential. Partial differentials are denoted as follows:

The sum of partial differentials for all independent variables gives the total differential. For a function of two independent variables, the total differential is expressed by the equality

(7)

Example 9. Find the complete differential of a function

Solution. The result of using formula (7):

A function that has a total differential at every point of a certain domain is said to be differentiable in that domain.

Find the total differential yourself and then look at the solution

Just as in the case of a function of one variable, the differentiability of a function in a certain domain implies its continuity in this domain, but not vice versa.

Let us formulate without proof a sufficient condition for the differentiability of a function.

Theorem. If the function z= f(x, y) has continuous partial derivatives

in a given region, then it is differentiable in this region and its differential is expressed by formula (7).

It can be shown that, just as in the case of a function of one variable, the differential of the function is the main linear part of the increment of the function, so in the case of a function of several variables, the total differential is the main, linear with respect to the increments of independent variables, part of the total increment of the function.

For a function of two variables, the total increment of the function has the form

(8)

where α and β are infinitesimal at and .

Higher order partial derivatives

Partial derivatives and functions f(x, y) themselves are some functions of the same variables and, in turn, can have derivatives with respect to different variables, which are called partial derivatives of higher orders.

Definition 1.11 Let a function of two variables be given z=z(x,y), (x,y)D . Dot M 0 (x 0 ;y 0 ) - internal point of the area D .

If in D there is such a neighborhood U.M. 0 points M 0 , which for all points

then point M 0 is called a local maximum point. And the meaning itself z(M 0 ) - local maximum.

And if for all points

then point M 0 is called the local minimum point of the function z(x,y) . And the meaning itself z(M 0 ) - local minimum.

The local maximum and local minimum are called local extrema of the function z(x,y) . In Fig. 1.4 explains the geometric meaning of the local maximum: M 0 - maximum point, since on the surface z =z (x,y) its corresponding point C 0 is higher than any neighboring point C (this is the locality of the maximum).

Note that there are generally points on the surface (for example, IN ), which are located above C 0 , but these points (for example, IN ) are not "neighboring" to the point C 0 .

In particular, point IN corresponds to the concept of global maximum:

The global minimum is defined similarly:

Finding global maxima and minima will be discussed in section 1.10.

Theorem 1.3(necessary conditions for an extremum).

Let the function be given z =z (x,y), (x,y)D . Dot M 0 (x 0 ;y 0 D - local extremum point.

If at this point there are z" x And z" y , That

The geometric proof is "obvious". If at the point C 0 draw a tangent plane on (Fig. 1.4), then it will “naturally” pass horizontally, i.e. at an angle 0° to the axis Oh and to the axis OU .

Then, in accordance with the geometric meaning of partial derivatives (Fig. 1.3):

which was what needed to be proven.

Definition 1.12.

If at the point M 0 conditions (1.41) are satisfied, then it is called a stationary point of the function z(x,y) .

Theorem 1.4(sufficient conditions for an extremum).

Let it be given z =z (x,y), (x,y)D , which has second-order partial derivatives in some neighborhood of the point M 0 (x 0 ,y 0 )D . Moreover M 0 - stationary point (i.e., the necessary conditions (1.41) are satisfied). Let's calculate:

The proof of the theorem uses topics (Taylor's formula for functions of several variables and the theory of quadratic forms) that are not covered in this tutorial.

Example 1.13.

Explore to the extreme:

Solution

1. Find stationary points by solving system (1.41):

that is, four stationary points are found. 2.

by Theorem 1.4 at the point there is a minimum. Moreover

by Theorem 1.4 at the point

Maximum. Moreover

And you don’t need to look for anything: in our separate article we have already prepared everything so that you can do this. And now we will talk about partial derivatives.

Welcome to our telegram channel for useful newsletters and current student news.

Function of two or more variables

Before we talk about partial derivatives, we need to touch on the concept of a function of several variables, without which there is no point in a partial derivative. At school we are used to dealing with functions of one variable:

We previously considered derivatives of such functions. The graph of a function of one variable is a line on a plane: a straight line, a parabola, a hyperbola, etc.

What if we add another variable? You will get the following function:

It is a function of two independent variables x And y. The graph of such a function is a surface in three-dimensional space: a ball, hyperboloid, paraboloid or some other spherical horse in a vacuum. Partial derivative functions z X and Y respectively are written as follows:

There are also functions of three or more variables. True, it is impossible to draw a graph of such a function: this would require at least a four-dimensional space, which cannot be depicted.

First order partial derivative

Let's remember the main rule:

When calculating the partial derivative with respect to one of the variables, the second variable is taken as a constant. Otherwise, the rules for calculating the derivative do not change.

That is, the partial derivative is essentially no different from the ordinary one. So, keep before your eyes the table of derivatives of elementary functions and the rules for calculating ordinary derivatives. Let's look at an example to make it completely clear. Let's say we need to calculate the first-order partial derivatives of the following function:

First, let's take the partial derivative with respect to x, considering y to be an ordinary number:

Now we calculate the partial derivative with respect to the y, taking x as a constant:

As you can see, there is nothing complicated about this, and success with more complex examples is just a matter of practice.

Second order partial derivative

How is the second order partial derivative found? The same as the first one. To find second-order partial derivatives, you simply take the derivative of the first-order derivative. Let's return to the example above and calculate the second-order partial derivatives.

By gamer:

Partial derivatives of the third and higher orders do not differ in the principle of calculation. Let's systematize the rules:

1. When differentiating by one independent variable, the second is taken as a constant.
2. A second-order derivative is a derivative of a first-order derivative. Third order – derivative of the second order derivative, etc.

Partial derivatives and total differential functions

A common question in practical tasks is finding the total differential of a function. For a function of several variables, the total differential is defined as the principal linear part of the small total increment of the function relative to the increments of the arguments.

The definition sounds cumbersome, but with letters everything is simpler. Full differential A first order function of several variables looks like this:

Knowing how partial derivatives are calculated, there is no problem in calculating the total differential.

Partial derivatives are not such a useless topic. For example, second-order partial differential equations are widely used to mathematically describe real-life physical processes.

Here we have given only a general, superficial idea of ​​partial derivatives of the first and second order. Are you interested in this topic or have specific questions? Ask them in the comments and contact the experts of professional student services for qualified and emergency assistance in your studies. With us you will not be left alone with the problem!

The general principle of finding second-order partial derivatives of a function of three variables is similar to the principle of finding second-order partial derivatives of a function of two variables.

In order to find second-order partial derivatives, you must first find first-order partial derivatives or, in another notation:

There are nine second-order partial derivatives.

The first group is the second derivatives with respect to the same variables:

Or – the second derivative with respect to “x”;

Or – the second derivative with respect to “Y”;

Or – the second derivative with respect to “zet”.

The second group is mixed 2nd order partial derivatives, there are six of them:

Or - mixed derivative “by x igrek”;

Or - mixed derivative “by game x”;

Or - mixed derivative “with respect to x z”;

Or - mixed derivative “by zt x”;

Or - mixed derivative “with respect to igrek z”;

Or - mixed derivative "by zt igrek".

As in the case of a function of two variables, when solving problems, you can focus on the following equalities of second-order mixed derivatives:

Note: strictly speaking, this is not always the case. For mixed derivatives to be equal, the requirement of their continuity must be met.

Just in case, here are a few examples of how to correctly read this disgrace out loud:

- “two strokes have twice a game”;

– “de two y by de z square”;

– “there are two strokes in X and Z”;

- “de two y po de zet po de igrek.”

Example 10

Find all first and second order partial derivatives for a function of three variables:

.

Solution: First, let's find the first-order partial derivatives:

We take the found derivative

and differentiate it by “Y”:

We take the found derivative

and differentiate it by “x”:

The equality is fulfilled. Fine.

Let's deal with the second pair of mixed derivatives.

We take the found derivative

and differentiate it by “z”:

We take the found derivative

and differentiate it by “x”:

The equality is fulfilled. Fine.

We deal with the third pair of mixed derivatives in a similar way:

The equality is fulfilled. Fine.

After the work done, we can guarantee that, firstly, we have correctly found all the 1st order partial derivatives, and secondly, we have also correctly found the mixed 2nd order partial derivatives.

It remains to find three more partial derivatives of the second order; here, in order to avoid mistakes, you should concentrate your attention as much as possible:

Ready. I repeat, the task is not so much difficult as it is voluminous. The solution can be shortened and referred to equalities of mixed partial derivatives, but in this case there will be no verification. Therefore, it is better to spend time and find All derivatives (in addition, the teacher may require this), or, as a last resort, check the draft.

Example 11

Find all first and second order partial derivatives for a function of three variables

.

This is an example for you to solve on your own.

Solutions and answers:

Example 2:Solution:

Example 4:Solution: Let's find the first order partial derivatives.

Let's create a first order complete differential:

Example 6:Solution: M(1, -1, 0):

Example 7:Solution: Let us calculate the first order partial derivatives at the pointM(1, 1, 1):

Example 9:Solution:

Example 11:Solution: Let's find the first order partial derivatives:

Let's find the second order partial derivatives:

.

Integrals

8.1. Indefinite integral. Detailed sample solutions

Let's start studying the topic " Indefinite integral", and we will also analyze in detail examples of solutions to the simplest (and not so simple) integrals. As usual, we will limit ourselves to the minimum of theory, which is in numerous textbooks; our task is to learn how to solve integrals.

What do you need to know to successfully master the material? In order to cope with integral calculus, you need to be able to find derivatives at a minimum, at an intermediate level. It will not be a waste of experience if you have several dozen, or better yet, hundreds of independently found derivatives under your belt. At the very least, you should not be confused by tasks to differentiate the simplest and most common functions.

It would seem, what do derivatives have to do with it if the article is about integrals?! Here's the thing. The fact is that finding derivatives and finding indefinite integrals (differentiation and integration) are two mutually inverse actions, such as addition/subtraction or multiplication/division. Thus, without skill and any experience in finding derivatives, unfortunately, you cannot move forward.

In this regard, we will need the following teaching materials: Derivatives table And Table of integrals.

What is the difficulty in learning indefinite integrals? If in derivatives there are strictly 5 rules of differentiation, a table of derivatives and a fairly clear algorithm of actions, then in integrals everything is different. There are dozens of integration methods and techniques. And, if the integration method is initially chosen incorrectly (i.e. you don’t know how to solve), then you can “prick” the integral literally for days, like a real puzzle, trying to spot various techniques and tricks. Some people even like it.

By the way, we quite often heard from students (not majoring in the humanities) an opinion like: “I’ve never had any interest in solving a limit or derivative, but integrals are a completely different matter, it’s fascinating, there’s always a desire to “hack” a complex integral.” . Stop. Enough of the black humor, let's move on to these very indefinite integrals.

Since there are many ways to solve it, then where should a teapot start studying indefinite integrals? In integral calculus, in our opinion, there are three pillars or a kind of “axis” around which everything else revolves. First of all, you should have a good understanding of the simplest integrals (this article).

Then you need to work through the lesson in detail. THIS IS THE MOST IMPORTANT TECHNIQUE! Perhaps even the most important article of all the articles on integrals. And thirdly, you should definitely read integration by parts method, since it integrates a wide class of functions. If you master at least these three lessons, then you will no longer have two. You may be forgiven for not knowing integrals of trigonometric functions , integrals of fractions, integrals of fractional-rational functions, integrals of irrational functions (roots), but if you “get into trouble” with the replacement method or the method of integration by parts, then it will be very, very bad.

So, let's start simple. Let's look at the table of integrals. As with derivatives, we notice several integration rules and a table of integrals of some elementary functions. Any table integral (and indeed any indefinite integral) has the form:

Let’s immediately understand the notations and terms:

– integral icon.

– integrand function (written with the letter “s”).

– differential icon. We will look at what this is very soon. The main thing is that when writing the integral and during the solution, it is important not to lose this icon. There will be a noticeable flaw.

– integrand expression or “filling” of the integral.

– antiderivative function.

– . There is no need to be heavily loaded with terms; the most important thing here is that in any indefinite integral a constant is added to the answer.

Solving an indefinite integral means findingmany primitive functions from the given integrand

Let's look at the entry again:

Let's look at the table of integrals.

What's happening? We have the left parts turn into to other functions: .

Let's simplify our definition:

Solve indefinite integral - this means TRANSFORM it into an undefined (up to a constant) function , using some rules, techniques and a table.

Take, for example, the table integral . What happened? Symbolic notation has evolved into many primitive functions.

As in the case of derivatives, in order to learn how to find integrals, it is not necessary to be aware of what an integral or antiderivative function is from a theoretical point of view. It is enough to simply carry out transformations according to some formal rules. So, in case It is not at all necessary to understand why the integral turns into . You can take this and other formulas for granted. Everyone uses electricity, but few people think about how electrons travel through wires.

Since differentiation and integration are opposite operations, for any antiderivative that is found correctly, the following is true:

In other words, if you differentiate the correct answer, then you must get the original integrand function.

Let's return to the same table integral .

Let us verify the validity of this formula. We take the derivative of the right side:

is the original integrand function.

By the way, it has become clearer why a constant is always assigned to a function. When differentiated, the constant always turns to zero.

Solve indefinite integral- it means to find a bunch of everyone antiderivatives, and not just one function. In the table example under consideration, , , , etc. – all these functions are solutions to the integral. There are infinitely many solutions, so we write it down briefly:

Thus, any indefinite integral is quite easy to check. This is some compensation for a large number of integrals of different types.

Let's move on to consider specific examples. Let's start, as in studying the derivative, with two rules of integration:

– constant C can (and should) be taken out of the integral sign.

– the integral of the sum (difference) of two functions is equal to the sum (difference) of two integrals. This rule is valid for any number of terms.

As you can see, the rules are basically the same as for derivatives. Sometimes they are called linearity properties integral.

Example 1

Find the indefinite integral.

Perform check.

Solution: It is more convenient to convert it like.

(1) Apply the rule . We forget to write down the differential icon dx under each integral. Why under each? dx– this is a full-fledged multiplier. If we describe it in detail, the first step should be written like this:

.

(2) According to the rule we move all the constants beyond the signs of the integrals. Please note that in the last term tg 5 is a constant, we also take it out.

In addition, at this step we prepare roots and powers for integration. In the same way as with differentiation, the roots must be represented in the form . Move the roots and powers that are located in the denominator upward.

Note: Unlike derivatives, roots in integrals should not always be reduced to the form , and move the degrees up.

For example, - this is a ready-made table integral, which has already been calculated before you, and all sorts of Chinese tricks like completely unnecessary. Likewise: – this is also a table integral; there is no point in representing the fraction in the form . Study the table carefully!

(3) All our integrals are tabular. We carry out the transformation using a table using the formulas: , And

for a power function - .

It should be noted that the table integral is a special case of the formula for a power function: .

Constant C it is enough to add once at the end of the expression

(rather than putting them after each integral).

(4) We write the result obtained in a more compact form, when all powers are of the form

again we represent them in the form of roots, and we reset the powers with a negative exponent back into the denominator.

Examination. In order to perform the check, you need to differentiate the received answer:

Received the original integrand, i.e. the integral was found correctly. What they danced from is what they returned to. It's good when the story with the integral ends this way.

From time to time, there is a slightly different approach to checking an indefinite integral, when not the derivative, but the differential is taken from the answer:

.

As a result, we get not an integrand function, but an integrand expression.

Don't be afraid of the concept of differential.

The differential is the derivative multiplied by dx.

However, what is important to us is not the theoretical subtleties, but what to do next with this differential. The differential is revealed as follows: icon d we remove it, put a prime on the right above the bracket, add a factor to the end of the expression dx :

Received original integrand, that is, the integral was found correctly.

As you can see, the differential comes down to finding the derivative. I like the second method of checking less, since I have to additionally draw large brackets and drag the differential icon dx until the end of the check. Although it is more correct, or “more respectable” or something.

In fact, it was possible to remain silent about the second method of verification. The point is not in the method, but in the fact that we have learned to open the differential. Again.

The differential is revealed as follows:

1) icon d remove;

2) on the right above the bracket we put a stroke (denotation of the derivative);

3) at the end of the expression we assign a factor dx .

For example:

Remember this. We will need this technique very soon.

Example 2

.

When we find an indefinite integral, we ALWAYS try to check Moreover, there is a great opportunity for this. Not all types of tasks in higher mathematics are a gift from this point of view. It doesn’t matter that checking is often not required in control tasks; no one and nothing prevents you from doing it on the draft. An exception can be made only when there is not enough time (for example, during a test or exam). Personally, I always check integrals, and I consider the lack of checking as a hack job and a poorly completed task.

Example 3

Find the indefinite integral:

. Perform check.

Solution: Analyzing the integral, we see that under the integral we have the product of two functions, and even the exponentiation of an entire expression. Unfortunately, in the field of integral battle No good and comfortable formulas for integrating the product and the quotient as: or .

Therefore, when a product or quotient is given, it always makes sense to see if it is possible to transform the integrand into a sum? The example under consideration is the case when it is possible.

First, we will present the complete solution, comments will be below.

(1) We use the good old formula of the square of the sum for any real numbers, getting rid of the degree over common bracket. outside the brackets and applying the abbreviated multiplication formula in the opposite direction: .

Example 4

Find the indefinite integral

Perform check.

This is an example for you to solve yourself. The answer and complete solution are at the end of the lesson.

Example 5

Find the indefinite integral

. Perform check.

In this example, the integrand is a fraction. When we see a fraction in the integrand, the first thought should be the question: “Is it possible to somehow get rid of this fraction, or at least simplify it?”

We notice that the denominator contains a single root of “X”. One in the field is not a warrior, which means we can divide the numerator by the denominator term by term:

We do not comment on actions with fractional powers, since they have been discussed many times in articles on the derivative of a function.

If you are still perplexed by such an example as

and in no case does the correct answer come out,

Also note that the solution is missing one step, namely applying the rules , . Usually, with some experience in solving integrals, these rules are considered an obvious fact and are not described in detail.

Example 6

Find the indefinite integral. Perform check.

This is an example for you to solve yourself. The answer and complete solution are at the end of the lesson.

IN general case with fractions in integrals it is not so simple; additional material on integrating fractions of some types can be found in the article: Integrating Some Fractions. But, before moving on to the above article, you need to familiarize yourself with the lesson: Substitution method in indefinite integral. The point is that subsuming a function under a differential or variable replacement method is key point in the study of the topic, since it is found not only “in pure tasks on the replacement method,” but also in many other types of integrals.

Solutions and answers:

Example 2: Solution:

Example 4: Solution:

In this example we used the abbreviated multiplication formula

Example 6: Solution:

Method of changing a variable in an indefinite integral. Examples of solutions

In this lesson we will get acquainted with one of the most important and most common techniques that is used when solving indefinite integrals - the variable change method. Successful mastery of the material requires initial knowledge and integration skills. If you have a feeling of an empty, full kettle in integral calculus, then you should first familiarize yourself with the material Indefinite integral. Examples of solutions, where it is explained in an accessible form what an integral is and basic examples for beginners are analyzed in detail.

Technically, the method of changing a variable in an indefinite integral is implemented in two ways:

– Subsuming the function under the differential sign.

– Actually changing the variable.

Essentially, these are the same thing, but the design of the solution looks different. Let's start with a simpler case.